

In such cases, we apply a property of definite integral that says ∫ a c f(t) dt = ∫ a b f(t) dt + ∫ b c f(t) dt and we assume 'b' to be a random constant while applying this property. Here, both the limits involve the variable t.

When Both Limits Have VariablesĬonsider the integral ∫ t² t³ log (x 3 + 1) dx. If the upper limit is a constant and the lower limit is a variable, say d/dx ∫ x 2 t 3 dt then we rewrite (using the property of definite integral) as - d/dx ∫ 2 x t 3 dt and now its result using the above examples is -x 3. Note that here the lower limit should be a constant. This would reflect the fact that the derivative of an integral is the original function itself. Then by the first fundamental theorem of calculus, d/dx ∫ a x f(t) dt = f(x). I.e., d/dx ∫ a b f(t) dt = 0 When One of the Limits is a ConstantĬonsider a definite integral ∫ a x f(t) dt, where 'a' is a constant and 'x' is a variable. Thus, when both limits are constants, the derivative of a definite integral is 0. d/dx∫ a b f(x) dx = d/dx = 0 (as F(b) and F(a) are constants). Then by the second fundamental theorem of calculus, ∫ a b f(x) dx = F(b) - F(a) where F(x) = ∫ f(t) dt. When Both Limits are ConstantsĬonsider the definite integral ∫ a b f(x) dx where both 'a' and 'b' are constants.
Chain rule integration how to#
We will study how to find the derivative of an integral in each of these cases.
The lower limit is a constant and the upper limit is a variable. But here, the limits always don't need to be constants. Also, let us see how to evaluate definite integrals that don't match with any of the last two cases.Ī definite integral is of the form ∫ a b f(t) dt. Let us look into each of these cases in detail. I.e., d/dx ∫ a x f(t) dt = f(x) where 'a' is a constant and 'x' is a variable. The derivative of a definite integral where the lower limit is a constant and the upper limit is a variable is a function itself in terms of the given variable (upper bound). The derivative of a definite integral with constant limits is 0. The derivative of an indefinite integral of a function is the function itself. The derivative of a definite integral of a function is the function itself only when the lower limit of the integral is a constant and the upper limit is the variable with respect to which we are differentiating. But this is always true only in the case of indefinite integrals. Calculus has massive applications to physics, chemistry, biology, economics and many other fields.The derivative of an integral of a function is the function itself. Integration has many applications in the sciences and economics to find "totals.". Integral techniques are important for finding any integral that is not immediately set up as one that is recognizable. Since the integrals are indefinite in this problem, they can be checked by differentiating the potential answer choices. The most common expressions to substitute are the objects by themselves, for example, in the denominator, in the exponent, under the radical, or as an argument to another function. The reverse chain rule is really just u-substitution with some minor manipulation of the d u. Knowledge of u-substitution and comfort with algebraic manipulation are encouraged to ensure success on this exercise. The user is expected to make an appropriate substitution and simplification, then find the antiderivative using more basic integrals. Find the indefinite integral: This problem asks for the integral of a function. There is one type of problem in this exercise: 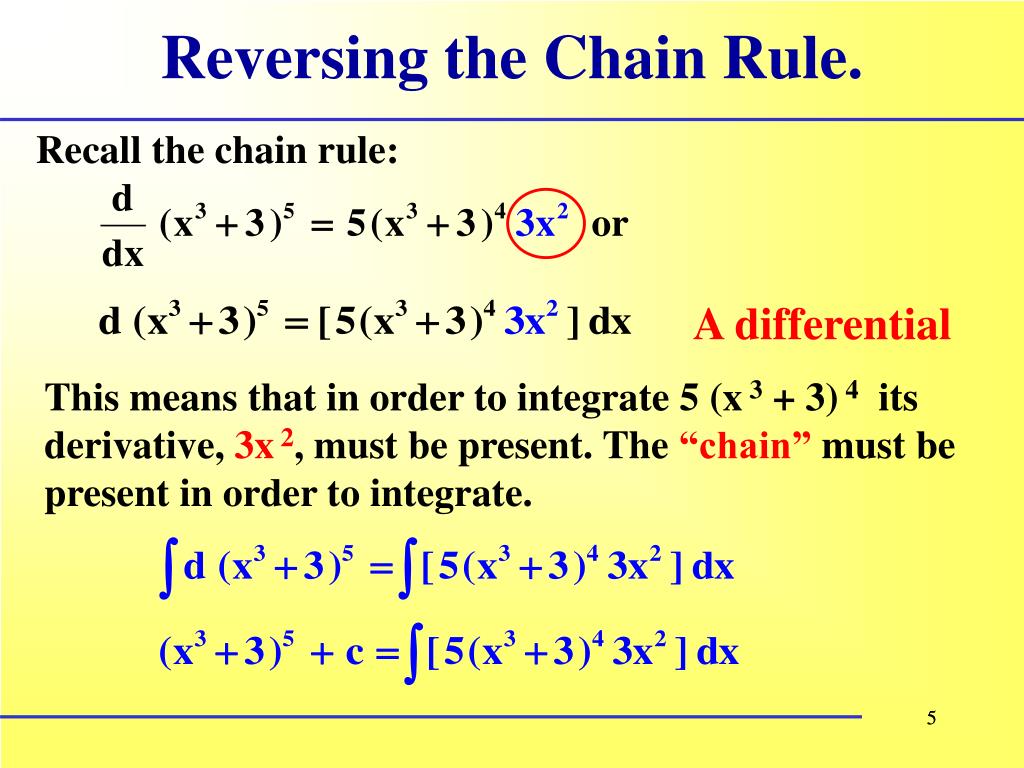
This exercise uses u-substitution in a more intensive way to find integrals of functions. The Integration by the reverse chain rule exercise appears under the Integral calculus Math Mission.







 0 kommentar(er)
0 kommentar(er)
